An Iterative Least Squares Method for Proton CT Image Reconstruction.
Presented by Don F. DeJongh and Ethan A. DeJongh
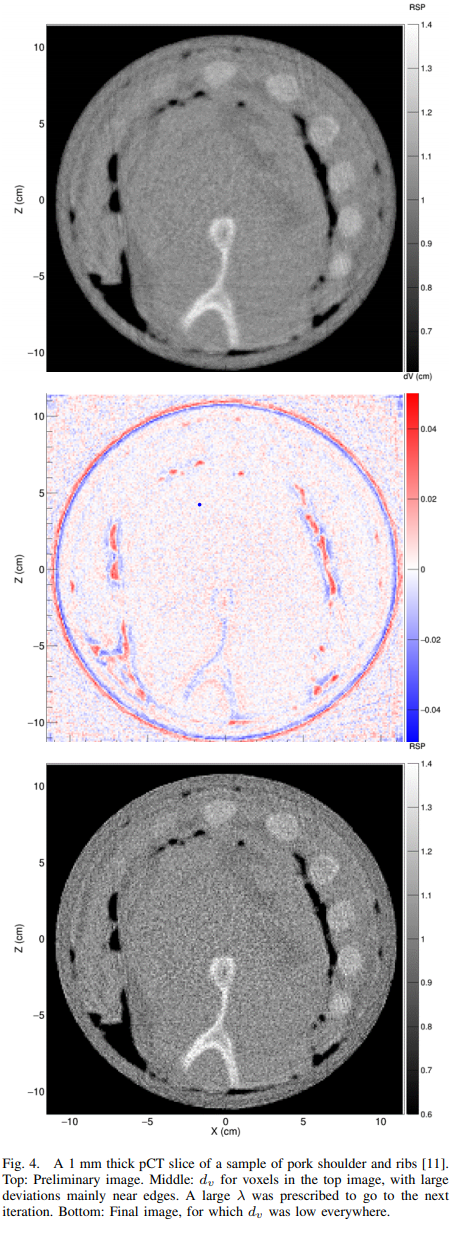
Abstract—Clinically useful proton Computed Tomography images will rely on algorithms to find the three-dimensional proton stopping power distribution that optimally fits the measured proton data. We present a least squares iterative method with many novel features to put proton imaging into a more quantitative framework. These include the definition of a unique solution that optimally fits the protons, the definition of an iteration vector that takes into account proton measurement uncertainties, the definition of an optimal step size for each iteration individually, the ability to simultaneously optimize the step sizes of many iterations, the ability to divide the proton data into arbitrary numbers of blocks for parallel processing and use of graphical processing units, and the definition of stopping criteria to determine when to stop iterating. We find that it is possible, for any object being imaged, to provide assurance that the image is quantifiably close to an optimal solution, and the optimization of step sizes reduces the total number of iterations required for convergence. We demonstrate the use of these algorithms on real data from the ProtonVDA system.